In this video series, we will show you the most common variants of the planar four-bar linkage, as well as their characteristics and special features. These variants can be derived from the theorem of Grashof.
Grashof’s condition for four-bar linkages states: The shortest link of a four-bar linkage can fully rotate in relation to its neighbouring links, if the sum of the lengths of the shortest and longest link is smaller – or at least equal, in the limit case – than the sum of the other two link lengths. The longest link in the four-bar linkage can be a neighbouring link of the shortest link or it can be situated opposite to it
According to the usual conventions for four-bar linkages, we name the links and joints as follows: The shortest link is always designated a, the remaining links are designated b, c and d in a clockwise direction.
For joints, the following applies, analogously: Joint 1 is between a and d and from there the further numbering up to 4 is also done clockwise.
Alternatively, the fixed link is usually referred to as the frame and the link opposite from it as the coupler. The other two links are often called grounded links.
By deciding which of the links b, c and d is the longest, as well as by different choices for the frame, different types of four-bar linkages can then be derived.
In this third part of our series, we will discuss the double-rocker. In contrast to crank-rocker and double-crank, it has only very few use cases. Again, we apply the Grashof condition, i.e. a+b < c+d, a+c < b+d or a+d < b+c, and we then apply our last remaining choice for the frame: the link opposite to the shortest link, c.
From the previous video on the double-crank we know that c cannot make full rotations relative to b and d. Conversely, b and d can only move back and forth within a limited range of angles. Thus, the linkage has two rockers, which explains its name.
Here, the lengths a to d were chosen as follows:
a = 45 (min), b = 160, c = 123, d = 161 (max)
Thus, the following applies: a+d = 45+161 = 206 < 283 = 160+123 = b+c
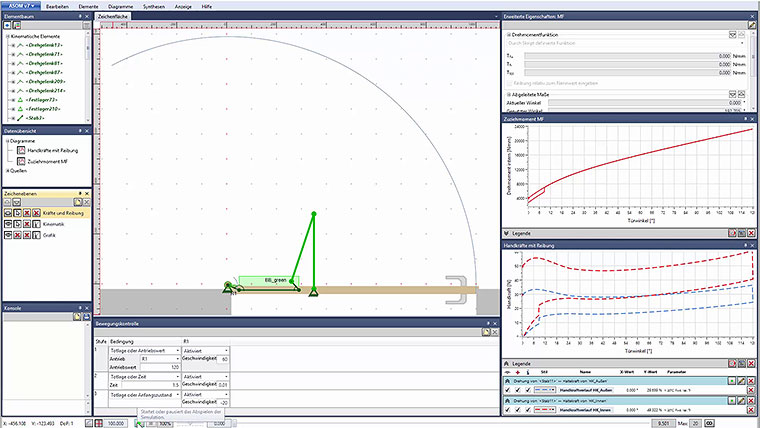
In ASOMmini, such linkages can be designed, simulated and analyzed kinematically very quickly and easily. They can also be modified and optimized in real time. The same also applies for more complex kinematic systems, which can also be implemented without difficulty using our software.